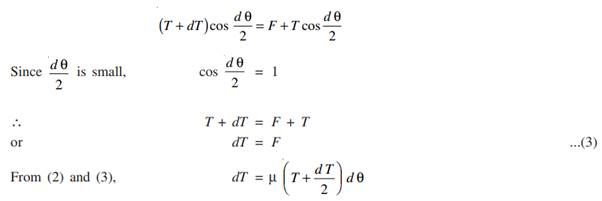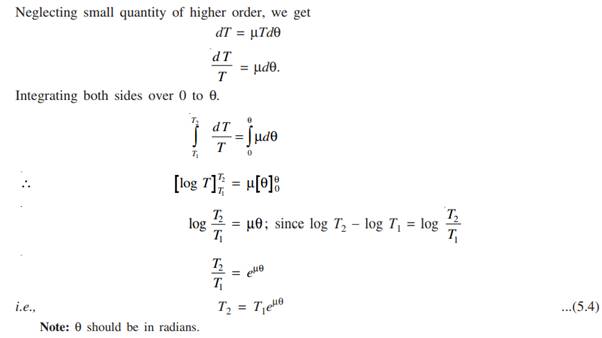The transmission of power by means of belt or rope drives is possible because of friction which exists between the wheels and the belt. Similarly, band brakes stops the rotating discs because of friction between the belt and the disc. All along the contact surface the frictional resistance develops. Hence, the tension in the rope is more on the side it is pulled and is less on the other side. Accordingly, the two sides of the rope may be called as tight side and slack side.

Relationship between Tight Side and Slack Side Forces in a Rope

Figure 5.17 (a) shows a load W being pulled by a force P over a fixed drum. Let the force on slack side be T1 and on tight side by T2 [Fig. 5.17(b)]. T2 is more than T1 because frictional force develops between drum and the rope [Fig. 5.17(c)]. Let θ be the angle of contact between rope and the drum. Now, consider an elemental length of rope as shown in Fig. 5.17(d). Let T be the force on slack side and T + dT on tight side. There will be normal reaction N on the rope in the radial direction and frictional force F = µN in the tangential direction. Then,