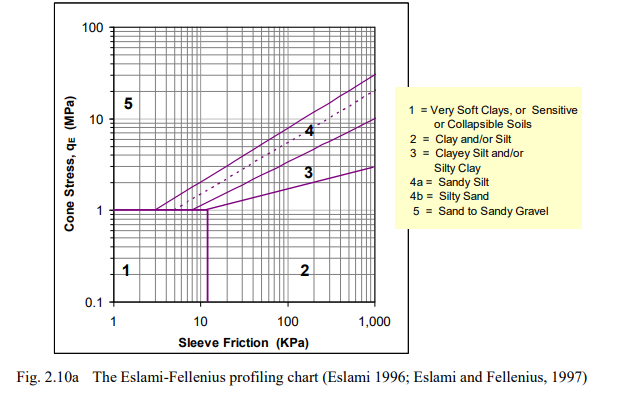
To investigate the use of cone penetrometer data in pile design, Eslami and Fellenius (1997) compiled a database consisting of CPT and CPTU data linked with results of boring, sampling, laboratory testing, and routine soil characteristics. The cases are from 18 sources reporting data from 20 sites in 5 countries. About half of the cases are from piezocone tests, CPTU, and include pore pressure measurements (U2). Non-CPTU tests are from sand soils and were used with the assumption that the U2-values are approximately equal to the neutral pore pressure (u0). The database values are separated on five main soil behavior categories as follows.

The data points were plotted in a Begemann type profiling chart and envelopes were drawn enclosing each of the five soil types. The envelopes are shown in Fig. 2.10a. The database does not include cases with cemented soils or very stiff clays, and, for this reason, no envelopes for such soil types are included in the chart.
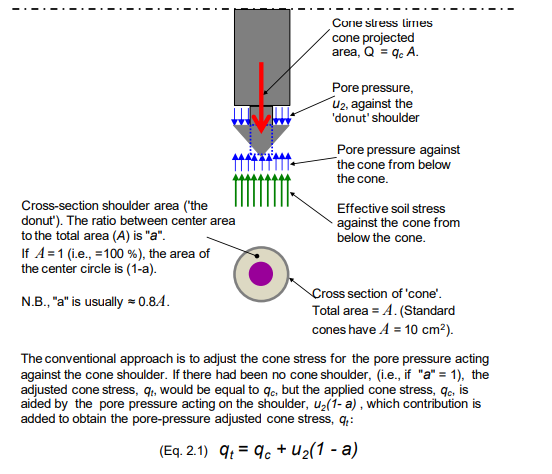
Plotting the “effective” cone stress, qE, defined by Eq. 2.6 is a filtering action (subtracting the pore pressure u2, which was found to provide a more consistent delineation of envelopes than a plot of only the pore pressure corrected stress, qt . Figure 10b shows the determining of the pore-pressure adjusted cone stress, qt , and the filtering by subtracting the pore pressure from qt .
The qE -value was also found to be a consistent value for use in relation to soil responses, such as pile shaft and pile toe resistances (Eslami 1996, Eslami and Fellenius 1995; 1996; 1997). Notice, However, that, as mentioned by Robertson (1990), the measured pore water pressure is a function of where the pore pressure gage is located. The pore pressure acting against the cone surface is not necessarily the same as the pore pressure acting on the cone shoulder. Therefore, the qE -value is not an effective stress attribute in the conventional sense. On the other hand, when evaluation of sounding data, e.g., records of dilatometer test (DMT), include adjusting the measured stress by subtracting the pore pressure.
Because the sleeve friction is a rather approximate measurement, no similar benefit was found in producing an “effective” sleeve friction. In dense, coarse-grained soils, the qE -value differs only marginally from the qt-value. In contrast, cone tests in fine-grained soils could generate substantial values of excess pore water pressure causing the qE -value to be much smaller than the qt-value, indeed, even negative, in which case the value should be taken as equal to zero.

The Eslami-Fellenius chart is simple to use and requires no adjustment to estimated value of the overburden stresses. The chart is primarily intended for soil type (profiling) analysis of CPTU data. With regard to the grain-size boundaries between the main soil fractions (clay, silt, sand, and gravel), international and North American practices agree. In contrast, differences exist with regard to how soiltype names are modified according to the contents of other than the main soil fraction. The chart assumes the name convention summarized in Section 1.3 as indicated in the Canadian Foundation Engineering Manual (1992, 2006).
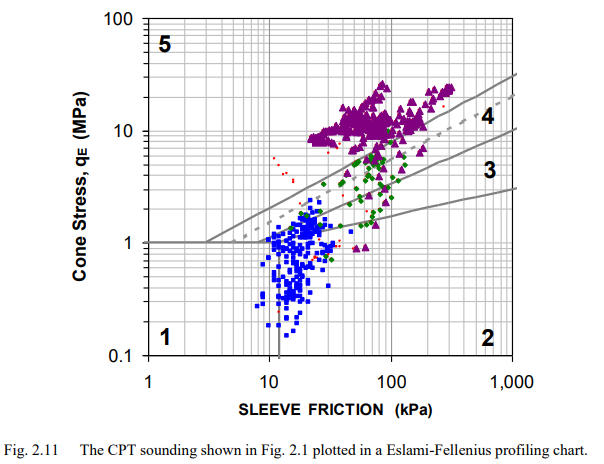
The data from the CPT diagrams presented in Fig. 2.1 are presented in the chart shown in Fig. 2.11. The three layers are presented with different symbols.