General
The Janbu approach, proposed by Nilmar Janbu in the early 1960s (Janbu 1963; 1965; 1967), and referenced by the Canadian Foundation Engineering Manual, CFEM (1985, 1992), combines the basic principles of linear and non-linear stress-strain behavior. The method applies to all soils, clays as well as sand. By the Janbu method, the relation between stress and strain is simply a function of two nondimensional parameters that are unique for any soil: a stress exponent, j, and a modulus number, m. (Strictly, the modulus is for constrained (“oedometer”) condition). Professor Janbu has presented a comprehensive summary of his method (Janbu 1998).
The Janbu approach is based on the definition of the conventional tangent modulus, Mt = ¶s/¶e, by the following expression (Eq. 3.7).

The Janbu expressions for strain are derived as follows.
Cohesionless Soil — j > 0
For cohesionless soil, the stress exponent is larger than zero, j > 0. Integrating Eq. 3.7 results in Eq. 3.8
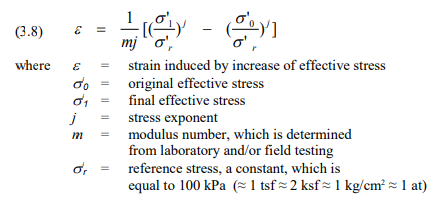
Mathematically, any stress exponent value larger than zero can be used. An exponent equal to unity indicates a linear stress-deformation response to load. A value smaller than unity agrees with the observation that for each increment stress the deformation of a soil volume becomes progressively smaller. A value larger than unity implies a soil where the incremental deformation increases with increasing stress. The latter has no practical application other than the fact that it, on occasion, can be useful in curve-fitting to observed records of stress or load versus movement, e.g., in dilative soil subjected to shear forces.
Dense Coarse-Grained Soil — j = 1
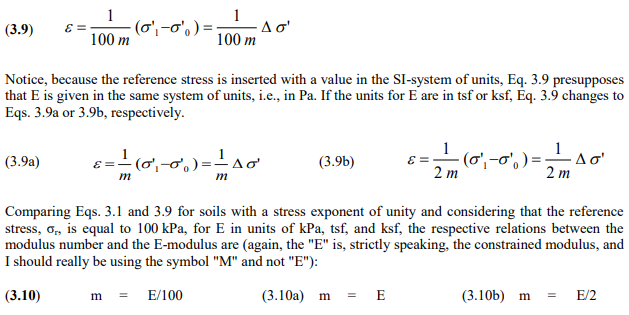
The stress-strain behavior (settlement) in dense coarse-grained soils, such as glacial till, can be assumed to be ‘elastic’, which means that the stress exponent is equal to unity (j = 1) and the compression is ‘linearly elastic”. It is normally assumed that immediate compression is linearly elastic, i.e., Ei and mi are constant and the stress exponent, j, is equal to unity. By inserting j = 1 and considering that the reference stress, σr , is equal to 100 kPa, Eq. 3.7 becomes Eq. 3.9.
Sandy or Silty Soil — j = 0.5
Janbu’s original concept considered a gradual increase of the stress exponent, j, from zero to unity when going from clay to dense gravel, though applying a gradual change is considered unnecessary in practice. Values of “j” other than j = 0 or j = 1, are only used for sandy or silty soils, where the stress exponent is often taken as equal to 0.5. (It actually reduces with decreasing grain size, but j = 0.5 is usually an acceptable average). By inserting this value and considering that the reference stress is 100 kPa, Eq. 3.7 simplifies to Eq. 3.11. For a soil, which is expected to be have a non-linear response (j = 0.5), where an average E-modulus is known for a range of stress, combining Eqs. 3.9 and 3.11 can enable a modulus number to be calibrated from (i.e., fitted to) a known E-modulus and stress range.
The Janbu modulus number can also be estimated from results of a CPTU sounding, as described in Section 2.11. This is an advantage of the Janbu approach because determining compressibility of coarsegrained soils in the laboratory is difficult.




